Visual receptive fields

Question

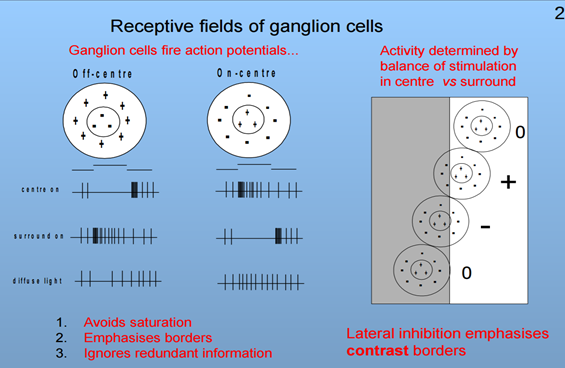
What I know about centre-surround type receptive fields is that depending on whether the region is on or off, the response to being stimulated is either excitatory or inhibitory respectively. So if a light shines on the centre of an on-centre/off-surround and off-centre/on-surround fields, spikes would be detected in the first case and not the second.

To my understanding, if both centre and surround areas are simultaneously stimulated, no spikes would be detected in either of the above-given fields. However, that means that if I am staring at what is essentially a blank white wall, I shouldn't be able to see anything. But that is obviously not happening. What am I missing here?

Also, on a related note - do on-centre & surround or off-centre & surround fields exist? What function do they serve, if any? What are the other types of visual receptive fields in humans, or other animals? Is there a major difference in properties of visual fields across species?

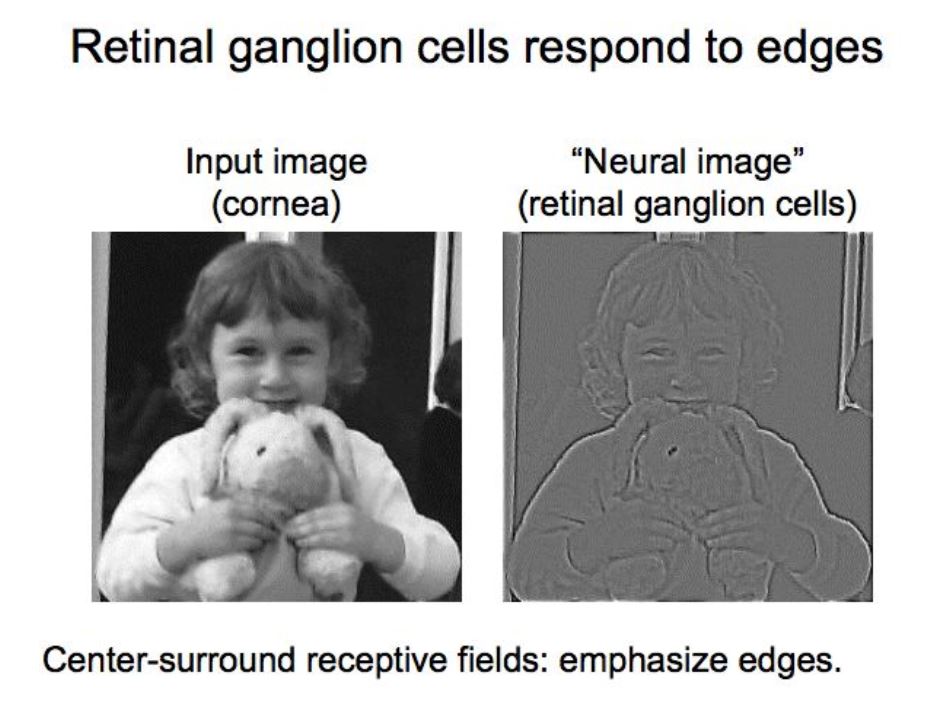
Edit: While browsing a few resources on receptive fields, I found this very-helpful image here.

This image perfectly illustrates my question: what happens to the grey areas in the right image? How are they processed by the retinal ganglion cells?

Answer 1

The centre + surround do not cancel completely, as you can see from these mudpuppy recordings. [Image 1] (Werblin and Dowling, 1969, I believe)

One function of a centre-surround set-up is to highlight the contrast at borders. Most of the useful visual information is from borders/outlines, so this is a method of ignoring redundant information. The highlighting of contrast borders and ignoring of redundant information explains why the neural image appears different. [Image 2 - right].

Another function of centre-surround fields is to avoid saturation, but this is more complicated to explain. [Image 2 - left] (Diagrams from Cambridge MVST Part IB lecture series by Hugh Matthews)