Can plants produce oxygen at night (without light)?

Question

I accidentally clicked on a "Top n X's that Y" result in google and found Top 9 Plants that gives (sic) off Oxygen at night as well (best for indoors).

In this helpful answer I've found a nice, concise description that I'm just going to reproduce here:

Photosynthesis consists of two general steps, one that requires light and one that does not. The first step occurs in the thylakoid membrane, and splits water into oxygen, hydrogen ions, and energy. The second step is the carbon fixation step, which occurs in the chloroplasts and uses energy to convert the carbon dioxide into carbohydrates.

Step 1: $\ce{2 H2O + light → 2 H+ + O2 + energy}$

Step 2: $\ce{3 CO2 + 6 H+ + energy → C3H6O3-P + 3 H2O}$

In general, the oxygen released from plants comes from water splitting which is driven (in a multi-step process) by photons. From what I remember, the oxygen molecules ($\ce{O2}$) are usually produced and released to the air within seconds, and certainly within minutes after all of the necessary photons for it have been absorbed.

So I am asking; are there plants that really make meaningful, or even just measurable amounts of oxygen without light?

(Or is the idea here that you leave your lights on at night and so actually any plant can make oxygen at night?)

Answer 1

Short Answer: Any plant can produce oxygen at night, but plants cannot produce oxygen without light.

Longer Answer: It all comes down to spontaneity of a reaction i.e. whether a reaction can occur without input of energy or not.

Before we talk about spontaneity, I feel it better to first know about the process we're talking about here. In photosynthesis, the water-splitting reaction occurs in the oxygen-evolving complex through a process known as Joliot-Kok cycle (or simply, Kok cycle)¹. Although, the complete mechanism of this process is not understood yet, the basic reaction can be depicted in a diagram as^2:

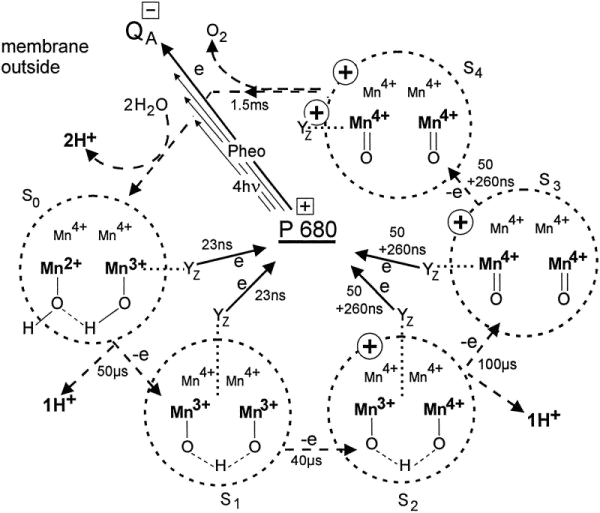
(Pay attention that in the diagram, the time taken is in microseconds and even nanoseconds. So, at molecular level, oxygen evolution does not take minutes or even seconds! However, at larger scale, release of oxygen might take longer time since the formed oxygen has to pass through many fluids, like thylakoid membrane, stroma, chloroplast membrane, cytoplasm, etc. before finally coming out in atmosphere)

To know where the photons are required (since the above diagram doesn't talk about that), I'll let in another diagram involving the steps where photons come in (as depicted by Rutherford et al, 1989):

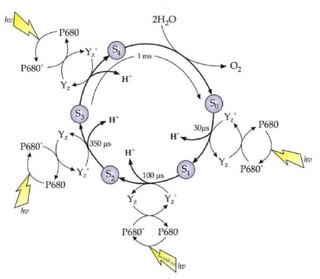
As you can see, Kok cycle does not get direct input of energy through photons. Rather, it is the high energy P₆₈₀+ which oxidizes a redox active tyrosine (Y_Z), which drives the reaction forward (the actual process is much more complex, see another answer or Halverson et al, 2003 for details).

Now, talking about spontaneity of this reaction, oxidation of water into hydrogen and oxygen is an endothermic process i.e. it is non-spontaneous in nature. In fact, the process requires 475 kJ/mol energy for occurring (Wikipedia). This energy barrier is so large that in the Kok cycle too, 4 photons are required to bring about oxidation of 1 water molecule (generally, catalysts are used so that they bring down the energy barrier of a non-spontaneous reaction so that it can be performed under normal conditions). Nilsson et al, 1857 talks about free energy change ($\Delta G_0$) of the cycle. See the graph for free energy change:

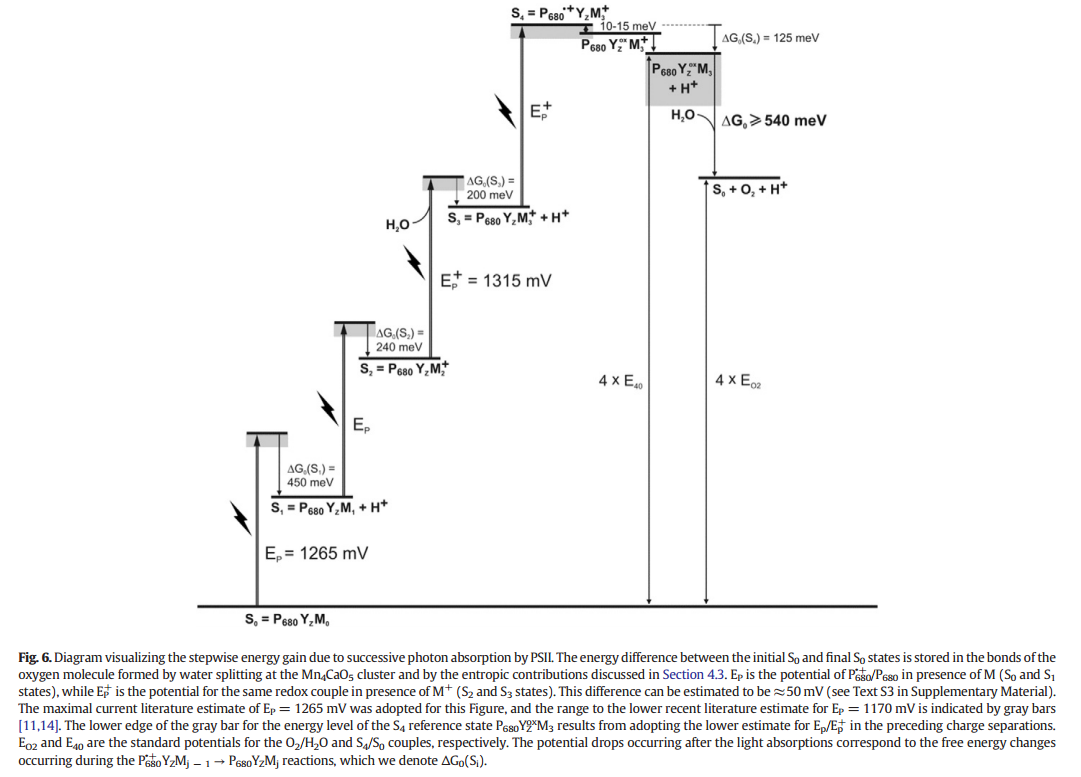
As is clearly visible from the graph, a higher amount of energy is needed to oxidize water ($E_P^+, S_2 \rightarrow S_3$) and even higher for liberation of dioxygen from the complex ($S_4 \rightarrow S_0$) (though the free energy change is negative for the last step i.e. liberation of oxygen is spontaneous, still the overall energy is larger than that at S₃). Thus, since the reaction requires overall energy input, plants cannot produce oxygen without light. Also, as you suspect already, the point here is the abundance of light from man-made sources which makes some plants able to produce oxygen at night.

BONUS: I'll add some details about the thermodynamics of the system (skip this part if you're not interested in maths). The basic equations I will use here are Gibbs Fundamental Equation ($\Delta G = \Delta H - T\Delta S$), Arrhenius Equation ($k = A \times e^{-E_a/RT}$) and Eyring Equation ($k = k_BT/h \times e^{-\Delta G^\ddagger/RT}$) and used data will be from Nilsson et al, 1857.

From $\Delta G = \Delta H - T\Delta S$, to make a reaction spontaneous ($\Delta G < 0$), either there should be release of heat ($\Delta H < 0$) or increase in entropy ($\Delta S > 0$). As we know now, the reaction is endothermic i.e. there is absorption of heat. So, there must be increase in overall entropy to drive the reaction forward. If you look at the graph for free energy change in different steps of reaction, it comes out to be like this:

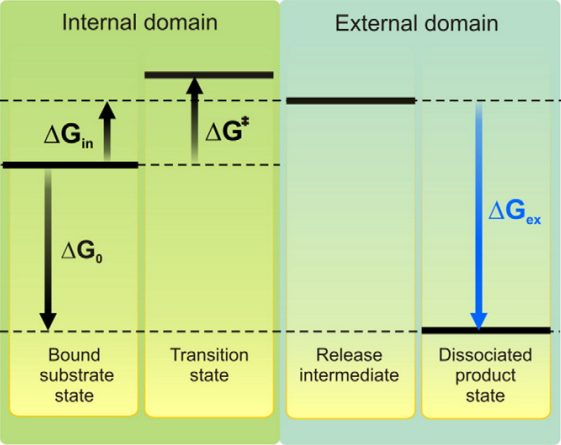
In this, $\Delta G_0$ is overall energy change, $\Delta G_{in}$ is the required energy input (given by photons), $\Delta G_{ex}$ is final free energy change and $\Delta G^\ddagger$ is Gibbs energy of activation. Here, $\Delta G_{ex}$ (shown in blue) corresponds to only the entropy change when final products (oxygen and protons) are released from the system as there is no enthalpy change here.

In release intermediate stage, the mole fraction $x = 1$ for oxygen, whereas in dissociated product stage mole fraction for water $x_1 = 56 /( [O_2]_0 + 56)$ and for oxygen $x_2 = [O_2]_0 / ([O_2]_0 + 56)$ (assuming molarity of water = 56 M) since in the final stage, water or oxygen or both can remain binded to the active site.

For release of oxygen: $[O_2]_0 = 273~\mu M$ and $T = 298~K$

which gives $\Delta G_1 = -~T\Delta S_1 = -~314~meV$

For release of proton: $\Delta G_2 = -~T\Delta S_2 = -~516~meV$ (since there is no enthalpy change, $\Delta H$ is neglected)

Now, $\Delta G_{ex} = \Delta G_1 + \Delta G_2 = -~314 - 516 = -~830~meV$

Also $\Delta G_0 = \Delta G_{in} + \Delta G_{ex}$

Since $\Delta G_{ex} > \Delta G_0$ therefore $\Delta G_{in} > 0$ i.e. energy is supplied.

Now, for the reaction $~~P_{680}Y_Z^{ox}M_3 \rightarrow P_{680}Y_ZM_0 + O_2$ (for which we've made the above graph)

Time constant $\tau \approx 10^{-3}~s$

i.e. $1/k = 10^{-3}~s$

or $k = 10^3~s^{-1}$

Using Eyring equation $k = k_BT/h \times e^{-\Delta G^\ddagger /RT}$

We get $\Delta G^\ddagger = -~580~meV$

In other words $\Delta G_{in} \leq -~580~meV$

Again $\Delta G_0 = \Delta G_{in} + \Delta G_{ex}$

On solving, $\Delta G_0 \leq -~250~meV$

Again, from Eyring equation, we get $k \geq 10^4~s^{-1}$

The large rate constant shows that there is a strong driving force for release of oxygen from the system, which is very likely due to increased entropy of the final product.

Thus, the enzyme brings down the free energy of the reaction, but at the expense of an increased activation energy barrier. Coming back to the main point, since energy is supplied to the system for the reaction, the reaction is non-spontaneous. However, the last step of the reaction is spontaneous (as also seen in the first graph) because of increase in translational and rotational entropy of final products when they are released (in above calculations, we neglected rotational entropy because of lack of data). Also, the above calculations were only for the last step of reaction ($S_4 \rightarrow S_0$) to show its spontaneity. In all other steps, energy has to be supplied to the system to drive the reaction forward (as shown in both first and second graphs above), making the overall reaction endergonic. I hope this helps now.

References:

_{1. Wikipedia contributors. "Oxygen-evolving complex." Wikipedia, The Free Encyclopedia. Wikipedia, The Free Encyclopedia, 1 Jan. 2017. Web. 18 Apr. 2017.}

_{2. Oxygen Evolution - 1996, Antony Crofts, University of Illinois at Urbana-Champaign}

_{3. A.W. Rutherford, Photosystem II, the water-splitting enzyme, Trends in Biochemical Sciences, Volume 14, Issue 6, 1989, Pages 227-232, ISSN 0968-0004, http://dx.doi.org/10.1016/0968-0004(89)90032-7.}

_{4. Halverson, Kelly M., and Bridgette A. Barry. “Evidence for Spontaneous Structural Changes in a Dark-Adapted State of Photosystem II.” Biophysical Journal 85.4 (2003): 2581–2588. Print.}

_{5. Wikipedia contributors. "Heterogeneous water oxidation." Wikipedia, The Free Encyclopedia. Wikipedia, The Free Encyclopedia, 18 Apr. 2017. Web. 18 Apr. 2017.}

_{6. Håkan Nilsson, Laurent Cournac, Fabrice Rappaport, Johannes Messinger, Jérôme Lavergne, Estimation of the driving force for dioxygen formation in photosynthesis, Biochimica et Biophysica Acta (BBA) - Bioenergetics, Volume 1857, Issue 1, January 2016, Pages 23-33, ISSN 0005-2728, http://doi.org/10.1016/j.bbabio.2015.09.011.}