Understanding Hodgkin-Huxley's model and activation variables

Question

This question is about the Hodgkin-Huxley model as introduced in Eugene M. Izhikevich, Dynamical Systems in Neuroscience, p.33 ff.

---

I'm having trouble to understand and interpret the differential equation for the activation variable $m$:

$$\dot{m} = (m_\infty(V) - m)/\tau(V)$$

which enters via

$$p = m^a h^b$$

into the equation

$$I = \bar{g} p (V − E)$$

for the net current $I$ generated by a large population of identical channels where $p$ is the average proportion of channels in the open state, $\bar{g}$ is the maximal conductance of the population, and $E$ is the reverse potential of the current.

$m$ is the probability of one of $a$ activation gates to be open. Interchangeably: $m^a$ is the proportion of open activation channels (assuming all of its activation gates must be open simultaneously).

The differential equation might give us $m$ as an explicit function of time but it – explicitly – involves $V$ which is – implicitly – another function of time, which in turn depends on the number of open gates. Things are horribly complicated!

On the other hand — since it's about voltage-gated channels and there are "immediate" voltage-sensitive regions in the channel protein which presumably don't have memory ("the single channel has no memory about the duration of its own state"), but possibly a time lag — I expected the being-open-probability of an activation gate to be a "pure" (possibly time-lagged) function of $V$.

My question comes in two disguises

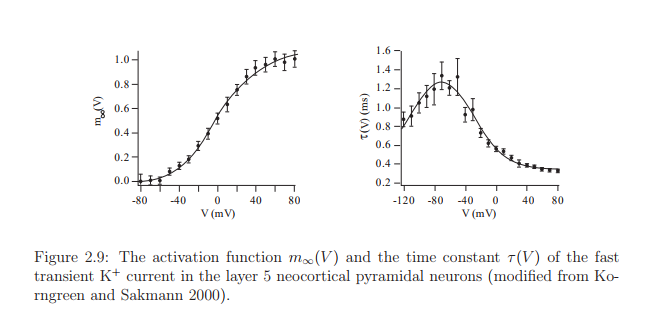
(1) Given two explicit functions $m_\infty(V)$ and $\tau(V)$ like these:

together with $m(0)$ and $V(0)$, how could we ever arrive at an explicit solution for $m(t)$, assuming that $V(t)$ depends somehow on $m(t')$ for $t'\leq t$, but possibly also on some injected currents.

(2) How can an intuitive and sensible interpretation of the terms in

$$\dot{m} = (m_\infty(V) - m)/\tau(V)$$

be given? What does the time constant $\tau(V)$ and its dependence on $V$ mean? In which respect and by which hypothesised mechanism is the gate "faster", when $\tau$ is smaller? How can the voltage-sensitive steady-state activation function $m_\infty(V)$ be intuitively explained other than by "giving the asymptotic value of $m$ when the potential $V$ is fixed (voltage-clamp)" or by some complicated description of experiments to determine it? What does $m_\infty(V) - m$ mean, i.e. "the deviation of the current activation from the steady-state activation"?

Answer 1

1) In practice, no one attempts to obtain explicit solutions for m(t), especially considering the presence of many other ion channel species, nor is there any need to. The standard of art is to use numerical simulations with a sampling interval dT that is sufficiently smaller than any relevant time constants.

2) I'm not sure what intuitive and sensible interpretation you are looking for; it may not make sense to come up with an intuitive explanation for each and every subset of terms. The use of time constants implies that the system is modeled as a linear time invariant system at a given voltage.

What does the time constant τ(V) and its dependence on V mean? In which respect and by which hypothesised mechanism is the gate "faster", when τ is smaller?

The time constant is a scalar that describes the rate at which the system approaches some equilibrium. You could think of the changes in channel state as chemical reactions of the form:

Open <=> Closed

which has some equilibrium and some rate constant - both the rate constant and equilibrium conditions can be a function of voltage, as depicted in the figure you provide. The physical meaning of a rate constant being voltage dependent would be that the activation energy for the transition between states is a function of voltage; when the activation energy is lower, the reaction can progress more quickly (in either direction).

How can the voltage-sensitive steady-state activation function m∞(V) be intuitively explained other than by "giving the asymptotic value of mm when the potential V is fixed (voltage-clamp)" or by some complicated description of experiments to determine it?

That's exactly what it means, and the descriptions are not complicated: the steady state value for a reversible reaction is the point at which the number of units moving from open to closed would be the same as the number moving from closed to open, so on average you see no more change: that's what steady-state means.

What does m∞(V)−m mean, i.e. "the deviation of the current activation from the steady-state activation"?

Yes, "the deviation of the current activation from the steady-state activation" is accurate. The equation is an equation for the change of m, and the change of m is proportional to the "distance from equilibrium" - this is a characteristic of any process that follows linear time invariant dynamics. Note that there are other ways to write this equation that involve a "forward rate", a "backward rate", and the concentration of units in each state (the Wikipedia Hodgkin-Huxley page you linked has these equations), but you can simplify these to the equation you show here because some proportion of the "forward rate" process is cancelled by the "backward rate": your equation is sufficient to describe the net change.