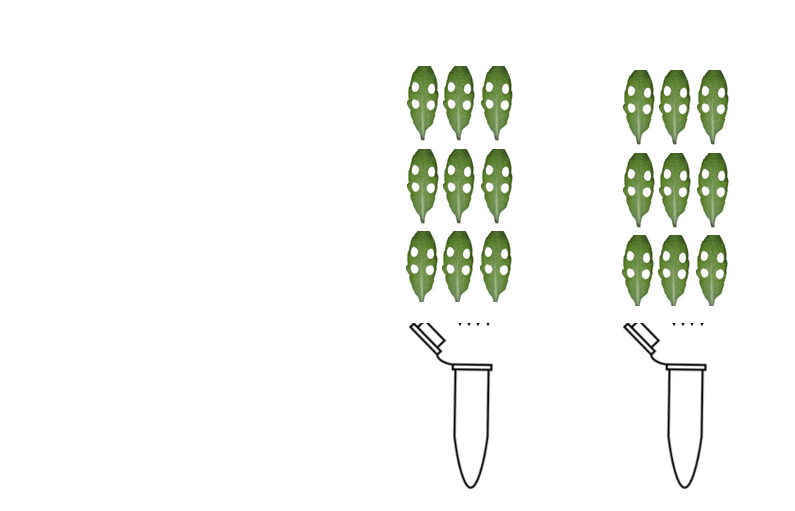
Your intuition is largely correct here. Bryan Krause's comments focus on where your answer is wrong. Some of his statements are true, others are speculative. His answer, combined with your intuition, could improve your measurements. I've shown a pooling scheme below. It should address the key ideas from both your question and Bryan's reply. The two key ideas here are (i) that pooling can improve estimates and (ii) that repeated measurements can give you insight into sampling variation.
Each observation you make will show a different amount of the phenolic compound. Some of this is due to actual differences in the amount of the phenolic compound. These differences can exist between fields of plants, between leaves on a plant, between punches from a single leaf. The list goes on. Furthermore, each measurement of a single sample produces a different reading. This is a lot to account for. If you would like to use statistics, then you should make it clear how your sample relates to the population you are interested in.
You mentioned that taking leaf punches is relatively easy. Pooling leaf samples uses that to your advantage. Let's say you will only be making three measurements for cultivar A and three measurements for cultivar B. For the first measurement you could grow 9 plants of cultivar A, and take 4 punches from each of those plants. You mix these punches together and measure the abundance of your phenolic compound. For the second measurement on cultivar A, you could repeat this entire process using different plants. The difference in these two measurements reflects sampling variation. This is due to all the sources mentioned above, and others. Decreasing the number of plants you pool doesn't solve the problem of sampling variation.
From a statistical standpoint, pooling can allow us to average. Lets say punches $i = 1, 2, \ldots, n$ are taken from cultivar A. They are prepared as equal volume samples. The corresponding concentration of the phenolic compound in each sample is $A_i$. Mixing these solutions results in a sample with concentration $\bar A_n$. The formula for this concentration can be obtained from the concentration of each $A_i$ as follows $$ \bar A_n = \frac{1}{n} \sum_{i = 1}^n A_i $$ These concentrations would be different if we repeated the experiment with a new sample of punches. To capture this, we can treat them as random variables.
We would like to show that pooling decreases the variance of $\bar A_n$. To see when this can happen note $$ \text{Var}\left[\frac{1}{n} \sum_i A_i\right] = \frac{1}{n} \left(\frac{1}{n} \sum_i \text{Var}[A_i]\right) + \frac{2}{n^2} \sum_{i < j} \text{Cov}(A_i, A_j) $$ The leading factor of $\frac{1}{n}$ is a key term here. It has the potential to make the variance small when the number of punches $n$ is large. This highlights the benefit of pooling, and is consistent with your suggestion.
A pooling strategy. Each tube measures a mixture created using multiple punches. Each mixture of punches is measured in a single tube.