Relationship between mN and mg in vessel contraction studies?

Question

What is the relationship between mN and mg as the units involved to measure the changes in contraction? A tool most widely used is an instrument called myograph. In these exoeriments, either units of mg or mN is used without any mention of why. Note that the first two examples use an experimental tool called a myograph - used to measure the force produced by a muscle (in this case muscular blood vessels) when under contraction. Both examples mention tension. Wether these myograph experiments are completely different i'm unsure.

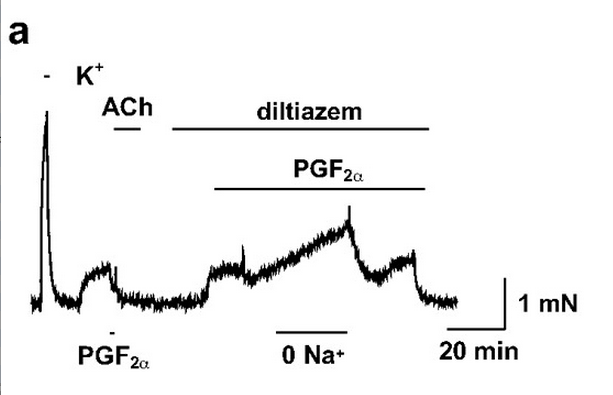
1. This example shows force as 'mN' from this paper Fig 1A a. Those who are interested the caption is as follows "Effects of Li+-PSS and NMDG-PSS in PGF2α-preconstricted pulmonary arteries. Intrapulmonary arteries (IPA) were first exposed to 80 mM K+, and endothelial function was assessed by relaxation of PGF2α contractions (10 μM) to acetylcholine (10 μM). Following a period of recovery, IPA were preconstricted to 10–15% 80K using PGF2α, and then physiological saline solution (PSS) was replaced by Li+-PSS in the continuing presence of the same concentration of PGF2α in endothelium-intact IPA"
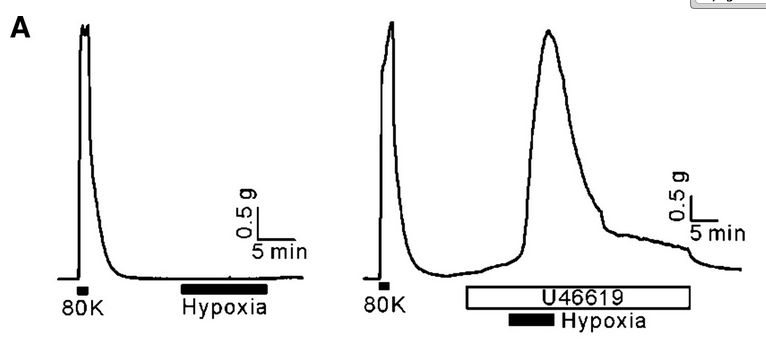
2. This example shows units as 'g'. note that some examples from other papers also use units of mg essentiall x1000 similar to other unit conversions from this paper Fig 1a. The caption is pretty much the same as 1. Particularly thromboxane A2 (TXA2) is a pharmacological agent affecting a set of ion channels: "Requirement of thromboxane A2 (TXA2) for hypoxic pulmonary vasoconstriction (HPV) in rat pulmonary arteries (PAs). A: hypoxia alone (3% PO2) did not induce contraction of PA (left). In the presence of 10 nM U46619, hypoxia induced a strong contraction equivalent with the response to high K+ (80K, right)"
3. For a third example click here. It does interestingly state "The PA rings were stretched to a predetermined optimal passive tension of 750 mg". As such mg (or g) is an alternative representation of a unit of force as presumed. Figure 1 in this link again demonstrates mg as an y-axis unit of measurement under myograph experiment

Answer 1

The difference between above two units is a matter of metric systems used: The newton (symbol: N) is the International System of Units (SI) derived unit of force. One newton is the force needed to accelerate one kilogram of mass at the rate of one metre per second squared.

Another system: kilogram (gram, milligram)-force The kilogram-force (kgf or kgF) is a gravitational metric unit of force. It is equal to the magnitude of the force exerted by one kilogram of mass in a 9.80665 m/s2 gravitational field (standard gravity, a conventional value approximating the average magnitude of gravity on Earth). Therefore one kilogram-force is by definition equal to 9.80665 N. Similarly, a gram-force is 9.80665 mN, and a milligram-force is 9.80665 µN. Kilogram-force is a non-standard unit and does not comply with the SI Metric System. See: http://www.sengpielaudio.com/ConvForce.htm