How are light sheet microscopy 3D images and their computed sections built up from stacks of 2D images?

Question

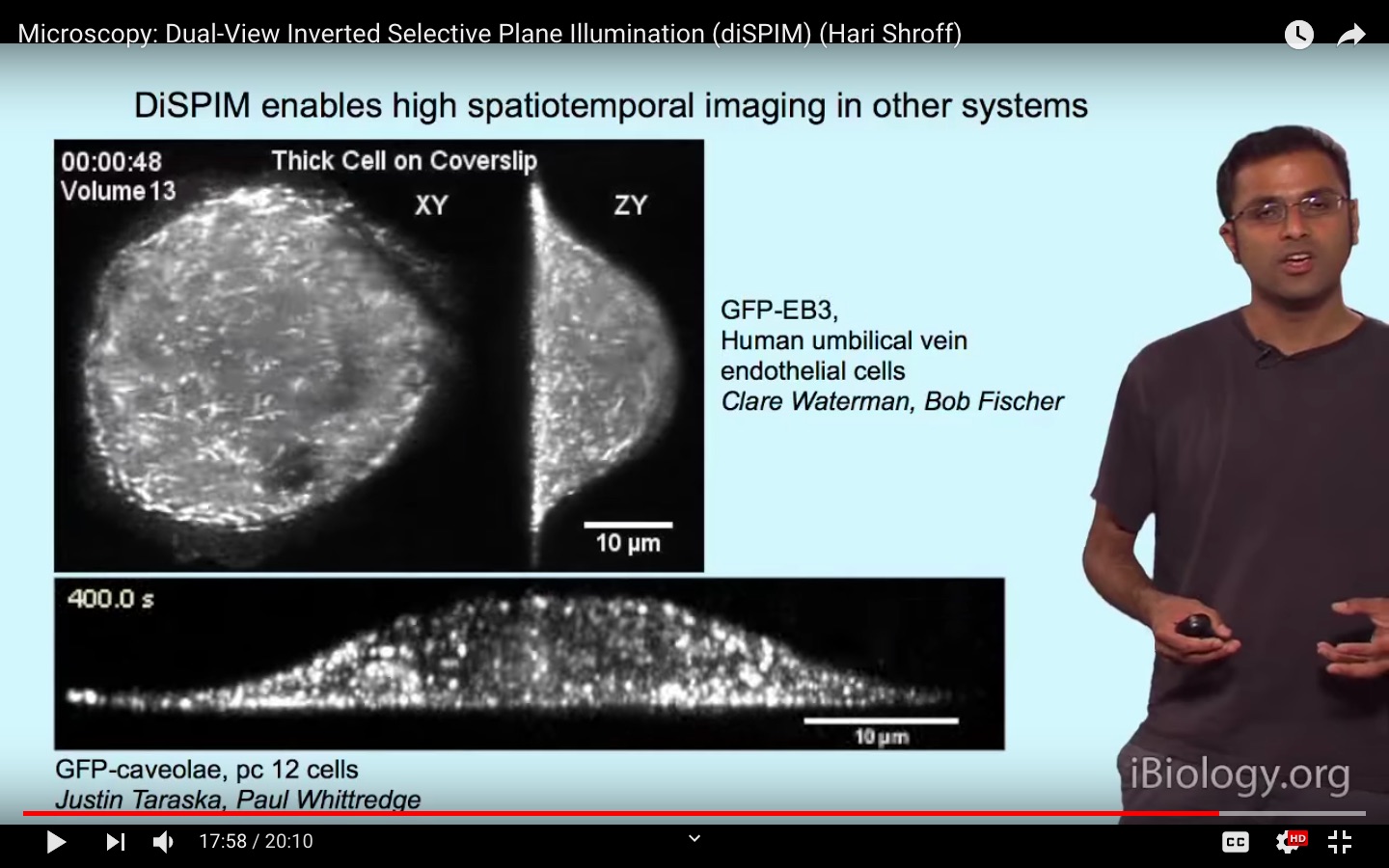
The iBiology Techniques video Microscopy: Dual-View Inverted Selective Plane Illumination (diSPIM) (Hari Shroff) describes light sheet microscopy and the improvement in resolution by introducing dual-view (two cameras) capability.

What's happening is that the object is moving randomly in 3D, and so for each frame, the stacks are used to generate a 3D image, and then it is de-rotated to synthesize a view in a plane fixed to the object, rather than the microscope.

Is there a simple way to understand how these image stacks are processed to obtain a smooth 3D reconstruction that can then be sectioned computationally? Is there some kind of interpolation or modeling of structure at the limit of the depth resolution?

Rather than a complete explanation, which would likely be fairly mathematical and in depth, a simpler explanation plus a reference or link to a more in-depth description might be a better way to help me get on the right track.

Answer 1

According to their website dispim.org they are using registration software like the ImageJ Multiview-Reconstruction Plugin. Judging from the descriptions, this plugin uses the alignment of multiple points in the different angle images (like fluorescent beads that have been introduced to the medium or the cell nuclei) by rigid transformation and then deconvolves the 3D information using the point spread functions.

Knowing your optical system (microscope lenses etc.) you can calculate how the image of a point light source would look like in the image. This can be used to reversely calculate the actual position of your light source from the image and reconstruct an image without the distortions.

From that they get a volumetric image that can be projected into any coordinate system. I recommend watching the videos on the plugin page to get an idea how this works.