About biological electrical potentials generally
Biological potentials on the order of tens of millivolts depend on very very few ions moving (about 1/100,000 of the potassium concentration, for example, for a typical neuronal potential).
Therefore, the sum of positive and negative charges in any compartment is always almost zero (to several decimal places and beyond measurement; if you say a ). Electrical forces are very powerful, and
Some previous answers that are related:
https://biology.stackexchange.com/a/57066/27148
https://biology.stackexchange.com/a/77925/27148
https://biology.stackexchange.com/a/81571/27148
The endocochlear potential in particular - this is really cool
Everything I said above basically can be summarized in a quick statement: you can't figure out potentials just by measuring ion concentrations and summing them up. If you counted all the ions (including charged proteins, etc), you'd get ~0 in any biological solution. Instead, you have to think about where the concentration gradients are and how particular ions are moving. Let's tackle the endocochlear potential... Hibino et al 2010 has an excellent diagram, adapted from their previous 2008 paper:
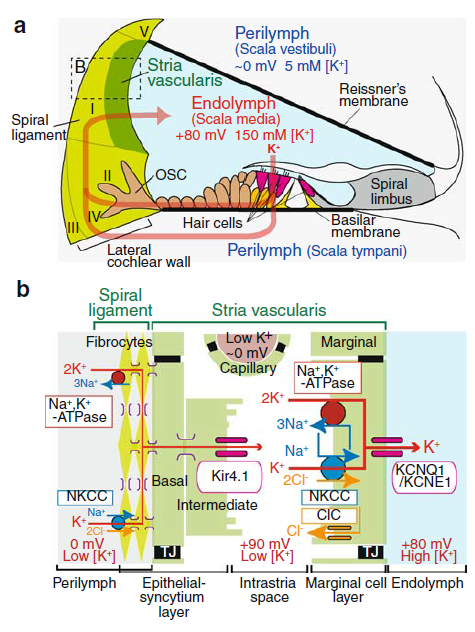
Let's walk through the key features here, focusing on panel (b). First, the general structure: there are three extracellular spaces, which are all electrically isolated from each other. Between these extracellular spaces, there are two layers of cells where all the business happens.
Between the perilymph and intrastria space, there are a collection of cell types all connected via gap junctions. We can treat them sort of like one big cell, I'll call it the syncytium. A sodium-potassium ATPase uses energy to pump potassium into the syncytium and sodium out (upper left of [b]). Additionally, because there is now low sodium in the syncytium, more potassium enters through NKCC channels, which are symporters that move 1 sodium, 1 potassium, and 2 chloride ions together.
Potassium would accumulate in the syncytium, except there are Kir4.1 channels permeable to potassium on the other side of these cells, open to the intrastria space. So, potassium flows down its concentration gradient into the intrastria space. This also creates an electrical potential and makes the intrastria space very positively charged (shown as +90mV on the diagram; the syncytium would similarly get a negative charge from the flowing potassium, but that isn't shown).
Normally, not many ions would actually flow to create this potential (on the order of the 1/100,000 I mentioned above) and everything would stop there. However, there are another set of pumps and channels in the marginal cells. The marginal cells also pump potassium in, via the sodium-potassium ATPase and NKCC channels, keeping potassium concentrations in the intrastria space low, such that potassium continues to flow down its concentration gradient from the syncytium.
The marginal cells also have potassium channels on their opposite face, and these are open to the endolymph. The high concentration of potassium ions in the marginal cells and the positive charge in the intrastria space gives the necessary drive to push the concentration of potassium in the endolymph high.
Ultimately, there is a full circuit of potassium because potassium is flowing into hair cells from the endolymph, and then back out to the perilymph. The sodium-potassium pumps use energy in the form of ATP to maintain the imbalance between perilymph and endolymph.
Hibino, H., Nin, F., Tsuzuki, C., & Kurachi, Y. (2010). How is the highly positive endocochlear potential formed? The specific architecture of the stria vascularis and the roles of the ion-transport apparatus. Pflügers Archiv-European Journal of Physiology, 459(4), 521-533.
Nin, F., Hibino, H., Doi, K., Suzuki, T., Hisa, Y., & Kurachi, Y. (2008). The endocochlear potential depends on two K+ diffusion potentials and an electrical barrier in the stria vascularis of the inner ear. Proceedings of the National Academy of Sciences, 105(5), 1751-1756.

