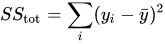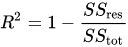0
$\begingroup$

Falconer's formula for broad sense heritability is 2*(r_monozygotic - r_dizygotic).

Mayhew and Meyer (2017) states: "Subtracting the DZ phenotypic correlation from the MZ phenotypic correlation provides an estimate of the variance contributed by genetics when 50% of DNA is shared."

If we are seeking an estimate of the additional variance explained by an additional 50% of shared genes, shouldn't we be subtracting r^2 values rather than r values because r^2 is the proportion of variance explained?

https://www.ncbi.nlm.nih.gov/pmc/articles/PMC5635617/

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Each of these two estimates (DZ correlation and MZ correlation) express a numerical relationship (Pearson correlation) between individuals. Each of these could independently be squared to provide r^2 values, if that were one's goal. And the final quantity (heritability, h^2) that Falconer's formula provides is analogous to a r^2. But that does not mean that all of the intermediate steps can be easily treated in terms of r^2.

I'll defer to wikipedia and other resources for more details, but in simple terms, variance (in r space) is easy to decompose into contributing factors (e.g. MZ correlation vs. DZ correlation), but r^2 is hard to decompose into contributing factors. See for example the general definition of r^2 given by wikipedia:

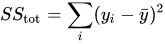
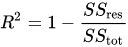
formula from wiki coefficient of determination article

formula from wiki coefficient of determination article

formula from wiki coefficient of determination article

When you are computing R^2, you don't sum (or subtract) variances explained by different factors. You compute residuals (differences like MZ vs DZ), square them, and sum them, and then say all the variance that isn't in that residual quantity must come from the model (e.g. is variance explained by the model).

I think one issue here is that people use terms like "variance explained" in slightly informal ways. It applies to both r^2 and the final heritability estimate, but not every intermediate step of the computation of those estimates can be neatly phrased in those terms.

For more background here, I can recommend the wiki page for heritability.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I'm suggesting subtracting r^2 values from each other. Not subtracting an r from an r^2. i.e. 2*(r^2_mz - r^2_dz) $\endgroup$
    – Ashish
    Commented Feb 9, 2022 at 20:42
  • $\begingroup$ @Ashish ok I misread your question. But the point about the decomposability of r vs. r^2 stands. I'll edit $\endgroup$
    – Maximilian Press
    Commented Feb 9, 2022 at 20:43
  • $\begingroup$ It is quite common to fit sequential linear regression models where you add a predictor with each model and report the difference in r^2 with each added IV. That difference represents the additional variance explained by the addition of the IV. Similarly, here you're adding 50% of the genome as a predictor. The difference in r^2 has a statistical meaning. Difference in correlations does not correspond to any clear statistical property as far as I can tell. My question is looking for a mathematical explanation of the substantive meaning of a difference in correlations. $\endgroup$
    – Ashish
    Commented Feb 10, 2022 at 18:31
  • $\begingroup$ @Ashish yes this is a common approach when performing regression analysis, one commonly evaluates models using this approach (though AIC or BIC are probably preferred for penalizing parameters). The r^2 difference is heuristically interesting for evaluating model fit in that case. It is however a different approach from what heritability estimation is trying to do, which is to try to estimate specific quantities of interest within a directly interpretable mathematical framework. $\endgroup$
    – Maximilian Press
    Commented Feb 10, 2022 at 18:58

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .