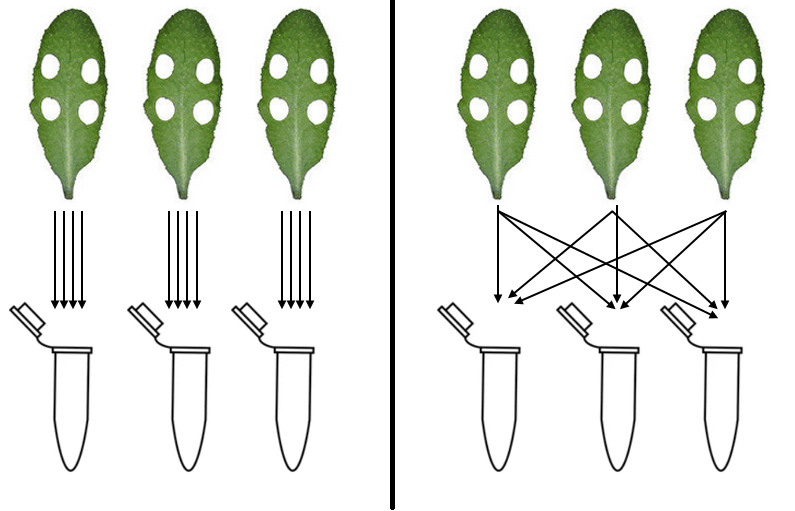
If you use the configuration on the left, your variance across tubes reflects variance between individuals.
If you use the configuration on the right, your variance across tubes reflects primarily variance in your assay (and perhaps variance within a leaf a bit).
Usually, you do an experiment like this because you'd like to be able to extrapolate your results to a larger population. To do that validly, you need to be able to estimate the variance in the population from your sample: the variance between individuals. Your configuration on the right will help you tell whether your samples are internally different, but they don't tell you much about what you can expect outside your sample. Importantly, one outlier plant would contaminate all the samples it contributes to.
Note that unless your effect sizes are expected to be very large, 3 individuals per group is likely an underpowered sample, meaning you do not have enough observations to detect a true difference in your groups. You cannot get around this by taking more samples from the same individuals, you need more individuals.
There are some cases where you might use an approach like on the right to test your assay, though not to draw conclusions about differences in treatments.
The language we use in statistics to describe these scenarios is independence. For, say, an unpaired t-test to be valid, the samples must be independent, both within and between groups (same is true for ANOVA equivalents with multiple groups). In the right scenario, your samples are not independent: every sample that includes part of "leaf 1" is going to have a relationship with every other sample that includes part of "leaf 1". If you treat those samples as if they are independent, you are breaking the assumption of your hypothesis tests and cannot rely on the results when comparing groups.
For a bit of an analogy that moves away from the more complicated biological assay part, let's say you want to know whether Group A is heavier than Group B. The procedure on the left is like weighing each member of Group A, and comparing these weights to members of Group B.
The procedure on the right is like having all members of Group A stand on a scale, then get off, then all stand on a scale again, then get off, etc. You'll have the best measurement you can get of how much the specific sample of Group A weighs across different measurements on your scale, but you won't be able to use your sample of Group A to estimate how much variation there is in Population A: your variation will just tell you how reliable your scale is. This is clearly a very very wrong procedure that is equivalent to the approach as you are proposing on the right-hand side of your question. Do not take this approach, you will waste your time and your results will be incorrect; if you hide the fact that you've taken this approach your work will be fraudulent.