Relation of conformational entropy and protein folding

Question

I'm trying to figure out the relation between conformational entropy and protein folding. I read the following in Lehninger, Principles of Biochemistry (6th edition):

Most of the net change in free energy as weak interactions form within a protein is therefore derived from the increased entropy in the surrounding aqueous solution resulting from the burial of hydrophobic surfaces. This more than counterbalances the large loss of conformational entropy as a polypeptide is constrained into its folded conformation.

In this context the meaning of conformational entropy is that the higher the stability of the proteïn is the lower the conformational entropy is (I think).

Why does the surrounding entropy increases because of the burial of hydrophobic residues?

Why does the burial of those hydrophobic residus couterbalances the large loss of conformational entropy?

I think maybe because the unfolded protein has more conformation then the protein when it's folded because of the hydorphobic core, it lowers the possible conformation because the hydrophobic interaction restrict the possiblities. Am I right?

Answer 1

This question is an example of an important general problem in chemical biology: “How does an ordered system arise without violating the second law of thermodynamics?” Berg et al., Biochemistry, addresses this in the following manner:

The Second Law of Thermodynamics states that the total entropy of a system and its surroundings always increases for a spontaneous process. At first glance, this law appears to contradict much common experience, particularly about biological systems. Many biological processes, such as the generation of a well-defined structure such as a leaf from carbon dioxide gas and other nutrients, clearly increase the level of order and hence decrease entropy. Entropy may be decreased locally in the formation of such ordered structures only if the entropy of other parts of the universe is increased by an equal or greater amount.

Your question is about the spontaneous folding of proteins. You ask:

In this context the meaning of conformational entropy is that the higher the stability of the proteïn is the lower the conformational entropy is (I think).

Why does the surrounding entropy increases because of the burial of hydrophobic residues?

Comments on your interpretation: Do not use the term ‘stability’ in this context. It is not a thermodynamic term and its meaning is imprecise. If we think of entropy as the degree of disorder or randomness, then we can see that Conformational entropy is used by the authors to mean the entropy of the protein as a random coil which can adopt many different structures. This has clearly a greater entropy than the folded protein with (to an approximation) a single structure. The adoption of the folded structure is thermodynamically favoured because it represents a state of lower energy (specifically Gibbs Free Energy) compared with the random coil structures, but entails a loss of entropy.

My answer: The surrounding entropy increases for the following reason. The surrounding entropy is that of the water molecules vibrating and interacting with other water molecules by means of the δ+ and δ– charges on H and O, respectively. In a system containing a protein with a random coil, the movement of these molecules is restricted by the hydrophobic side-chains they encounter, hence limiting the entropy, as it were. When the protein folds into a globule with polar residues on its surface, the vibrating water molecules are less restricted in their movement and hence have a higher entropy.

Further you ask:

Why does the burial of those hydrophobic residus couterbalances the large loss of conformational entropy?

I think maybe because the unfolded protein has more conformation then the protein when it's folded because of the hydorphobic core, it lowers the possible conformation because the hydrophobic interaction restrict the possiblities. Am I right?

My Answer: I have explained that in general terms above, in response to your first question. If you want a quantitative, mathematical justification you’ll be pushed to find one. Assuming that there are no other factors involved, we assume that it must be so because of the second law.

Comments on Your Answer: I don’t understand what you mean by “it lowers the possible conformation”. You can decrease the number of possible conformations — you cannot "lower possible conformation”. I assume English is your first language. You must try to use it more precisely if you are going to write about science (as well as checking your spelling, grammar and capitalization). If I were marking this paragraph as an exam answer I would put a line through it as unintelligible. The key thing is that you are comparing a single conformation of the lowest energy involving specific weak interactions and a polar surface, with a number of conformations which expose hydrophobic residues and restrict the free vibrations of the water molecules.

Further Clarification

The diagram below illustrates the problem:

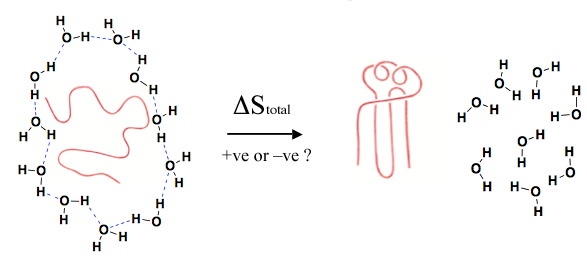
The protein is changing from a disordered to an ordered state so, its entropy is decreasing:

ΔS_protein = x , where x < 0

Whereas the water is changing from a more ordered to a less ordered state, so its entropy is increasing:

ΔS_water = y , where y > 0

and

ΔS_total = ΔS_protein + ΔS_water = x + y

If ΔS_protein + ΔS_water = 0 (x + y = 0), we would say that the increase in entropy of the water counterbalances the increase in entropy of the protein. For the folding to occur spontaneously, the total entropy must increase (ΔS_total > 0 , i.e. x + y > 0) and we would change the previous statement to “more than counterbalances”.