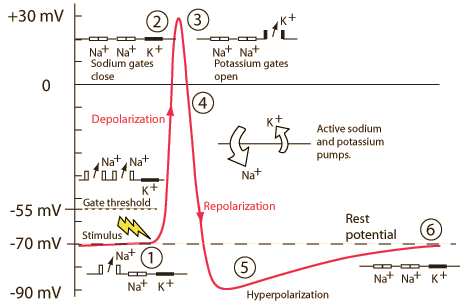
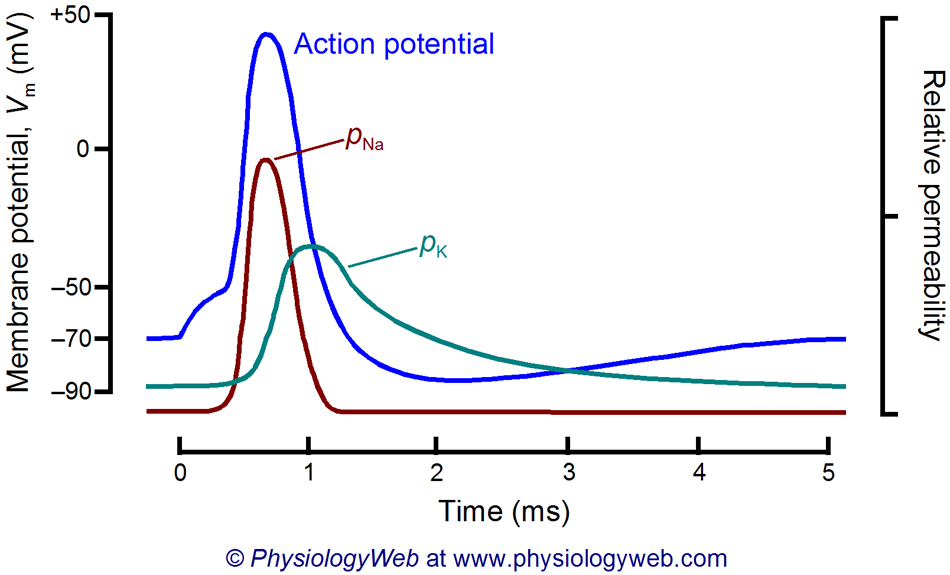
Very good question. I use it routinely when I teach basic neurophysiology. The key to the answer is to understand that the change in the membrane potential is not caused by transport of large amounts of ions over the membrane. It is simply the change in permeability of the membrane to these ions that change the potential. The membrane potential can be calculated by the Goldman equation from the permeabilities of the individual ions ($\ p_x$ indicates the permeability of ion X).
$\ E_m = \frac{RT}{F} \ln{ \left( \frac{ P_{\text{Na}}[\text{Na}^{+}]_\mathrm{out} + P_{\text{K}}[\text{K}^{+}]_\mathrm{out} + P_{\text{Cl}}[\text{Cl}^{-}]_\mathrm{in} }{ P_{\text{Na}}[\text{Na}^{+}]_\mathrm{in} + P_{\text{K}}[\text{K}^{+}]_{\mathrm{in}} + P_{\text{Cl}}[\text{Cl}^{-}]_\mathrm{out} } \right) }$
It is therefore not the transfer of charges as such that drive the change in membrane potential, but a small amount of ions must be moved to counteract the capacitive properties of the cell membrane. The cell membrane acts as a capactor, which means that the voltage across the cell membrane cannot change instantly, but changes as charges are redistributed between the two sides. These charges are moved by Na and K ions moving through the channels in the membrane.
Because we can model the membrane as a capacitor in an electric equivalent circuit, we can estimate the amount of ions moved during the AP. The charge (Q) across a capacitor (cell membrane) is measured in Coulomb (C) and calculated as the product of the capacitance and the voltage across the capacitor: $\ Q=C_m V_m$. If we assume a spherical cell with diameter 10µm and a membrane capacitance of 1$\mu F/cm^2 $ (= $\ 0.01F/m^2 $) and for simplicity assume that the membrane potential changes by 0.1V during an action potential (from -70 mV to +30 mV), we can calculate the number of charges moved.
The total capacity of the cell membrane is $\ 4\times\pi \times (5\times10^{-6}m)^2 \times 0.01F/m^2 = 3.14\times10^{-10}F $. As we change the voltage by 0.1V, this means that we must move a charge of $\ 3.14\times10^{-10}F\times0.1V=3.14\times10^{-11}C $ across the membrane. Given that the charge carried by one monovalent ion (the elementary charge) is $\ 1.6\times10^{-19}C$ we must move $\ 2\times10^6$ ions.
The volume of the cell is $\ \frac43\pi\times(5\times10^{-6}m)^3 =5.24\times10^{-16}m^3=5.24\times10^{-13}l$. If we assume intracellular concentrations at the start of the action potential to be [Na+] = 0.01M and [K+] = 0.15M we can calculate the number of Na+ and K+ ions in the cell by multiplying with Avogadro's number ($\ A_N = 6.02\times10^{23}$). Thus: number of Na+ ions = $\ 0.01M\times5.24\times10^{-13}l\times6.02\times10^{23}=3\times10^9$ and number of K+ ions = $\ 4.7\times10^{10}$.
Given that $\ 2\times10^6$ Na+ ions were moved into the cell during an AP and the same number of K+ ions moved out, the percent change in concentration can be calculated. The change in $\ [Na^+]$ due to one AP is therefore $\ \frac{2\times10^6}{3\times10^9}\times100\ percent=0.07\ percent $
and the change in $\ [K^+]$ is $\ \frac{4.7\times10^{10}}{3\times10^9}\times100\ percent=0.004\ percent$
In conclusion, the changes in intracellular concentrations of Na and K during a single action potential is insignficant, and can easily be counterbalanced by the continuous action of the Na/K-ATPase. In most cases, even if the nerve cell fires repeatedly at high rates, there is no measurable change in concentrations.