What is the biological limit on hearing resolution?

Question

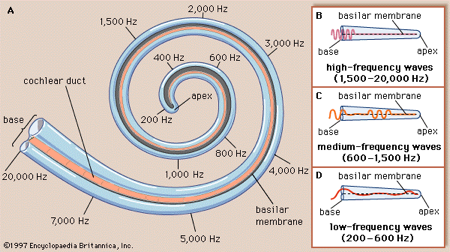
I sometimes wonder how many different individual musical scales could be perceived by human ears. I define a musical scale as a collection of notes that relate to some fundamental frequency by specific ratios. For instance

• do $2^{0}f$
• re $2^{\frac{1}{6}}f$
• mi $2^{\frac{1}{3}}f$
• fa $2^{\frac{5}{12}}f$
• sol $2^{\frac{7}{12}}f$
• la $2^{\frac{9}{12}}f$
• ti $2^{\frac{11}{12}}f$
• do $2^{1}f$

I once did an experiment where I took various sine waves at different frequencies and multiplied them by the appropriate ratios. I found for the ones I tried that I did indeed heard the major scale. But a mathematician will look at the positive real numbers $\Bbb{R}^+$ and say there are an infinite, uncountable number of possible frequencies. However, Biologically I cannot imagine the human ear is capable of distinguishing between an infinite number of frequencies.

What is the biological limit on hearing resolution? Is there a way to estimate how many different major scales one can hear? In other words, how many different frequencies can be heard?

Answer 1

Short answer
The main limitation to frequency discrimination is loudness.

Background
The cochlea is tonotopically organized - it basically acts as a Fourier transformer, where different frequencies are analyzed on a place map (Fig. 1). Frequency tuning of cochlear hair cells is mainly limited by sound intensity. The louder a pure-tone sound stimulus gets, the larger the area on the basilar membrane that is activated and hence the wider the range of cochlear hair cells that is activated, and hence the worse the frequency resolution becomes (Fig. 2).

^{Fig. 1. Cochlear tonotopy. Source: Cochlear Implant Help}

^{Auditory nerve fiber tuning. The louder the stimulus, the worse the tuning to frequency. Source: - Fettiplace & Hackney, 2006}

As to the quantitative aspect of this question, namely what the frequency difference limen is, and an indication how many frequency bands can be distinguished I refer you to below linked question. Both depend highly on the method used to determine the just-noticeable-difference, and as said also on the sound intensity applied. Moreover, it is dependent on the frequency under consideration. The higher the frequency, the larger the frequency band becomes expressed in Hz. This is an example of Weber-Fechner's law (Hecht, 1924). The cochlea is organized in octaves, meaning that higher frequencies have less cochlear space devoted to them and hence less hair cells to code a particular frequency range.

<sub>Further Readings
- What's the frequency resolution of the human ear?</sub>

<sub>Reference
- Hecht, J General Physiol (1924): 235-67
- Fettiplace & Hackney, Nature Rev Neurosci (2006); 7: 19-29</sub>