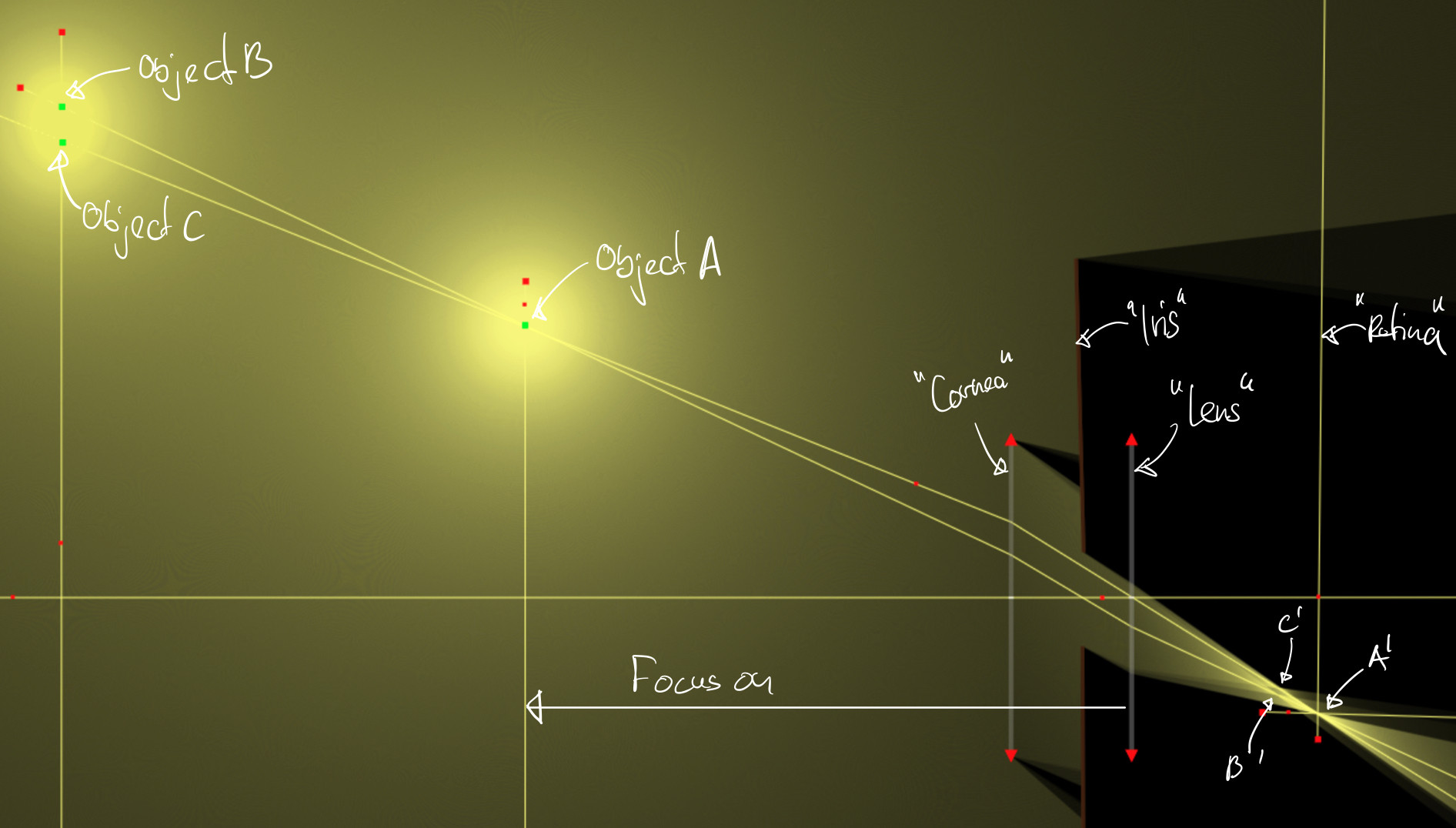
I read that the center of projection of the human eye is the entrance pupil. So given a light ray which intersects the objects position and the center of the aperture stop, all copies of said object when placed along that line should cover the exact same position/area on the retina (assuming they are scaled according to their distance).
However, doesn't this ignore the effect of accomodation of the human eye: If the original object is quite near, but a scaled copy along the ray is quite distant, the focal length of the lens is changed and thus a different position and area of the retina is covered.
I tried to visualize this by using the ray simulator at https://ricktu288.github.io/ray-optics/simulator/:
Image A:
Lens focussed on distance of Object A which is projected onto A' on the retina. Object copies B and C are placed at fixed distance either along a ray via the aperture stop (B) or via the center of the lens (C).
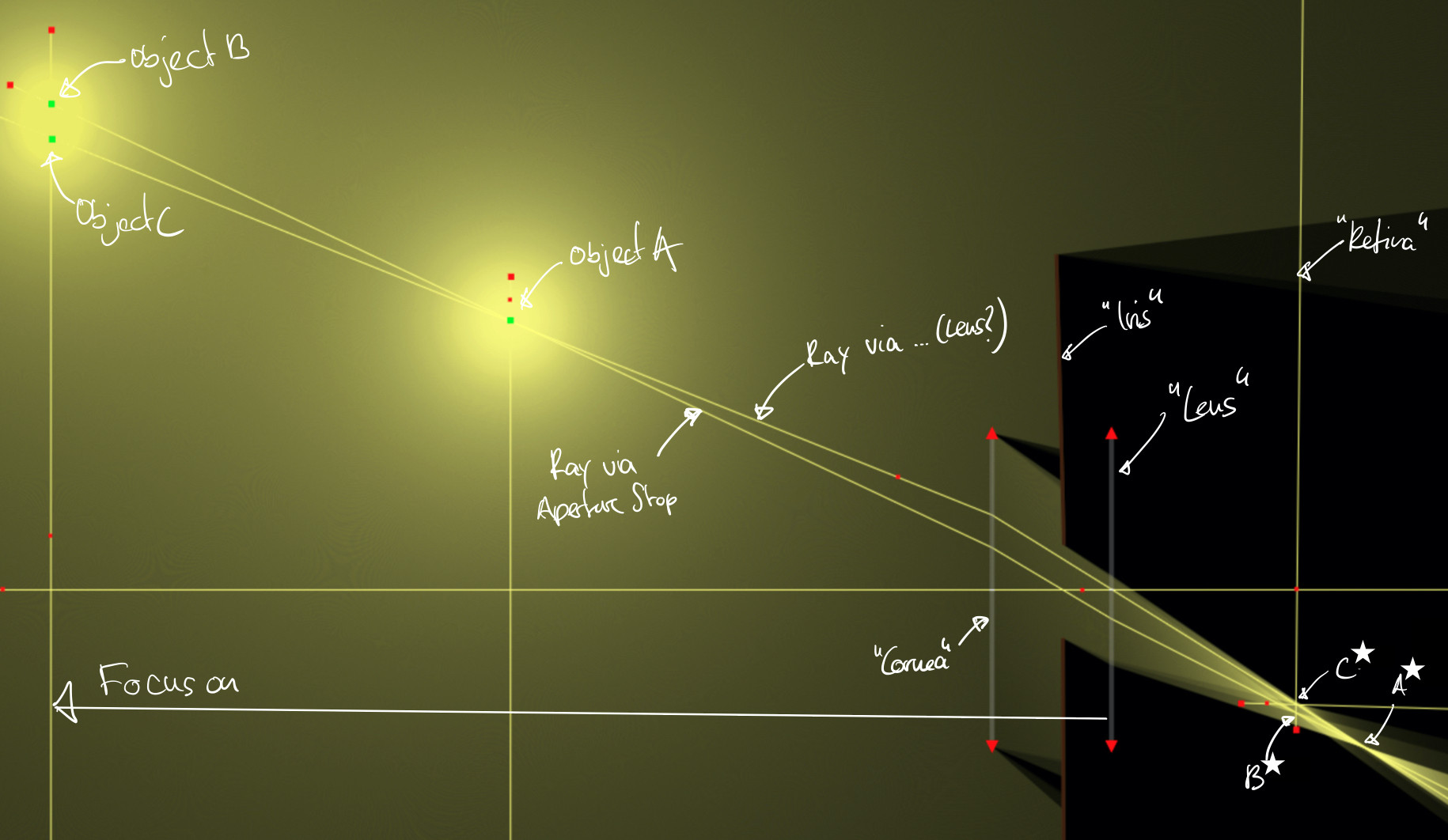
Image B:
Lens is focussed on distance of Object B/C which are projected onto B* and C* on the retina. Both are in focus (difficult to see in the picture), but B* is further away from A' than C*. Object B would appear larger than Object C.
So I guess this boils down to the question:
Given the position P of an object A and a lens system, what is the point Q which creates a ray PQ so that all scaled copies of A (A', A'', ...) at different distances along that PQ ray appear at the same position and area on the image created via the lens system.
Is there such a point Q? Is it the center of the lens (i.e. is C* == A') or one of its nodal points?
I am thankful for any pointer to help me understand this!