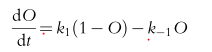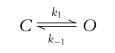How do you derive steady-state conditions from the open-state differential equation of ion channels?

Question

My books describes this differential equation for the open state of an ion channel:

Where $k$ are the rate constants,

$k_1$ denotes a forward reaction

$k_{-1}$ denotes a reverse reaction in the Markov model

$O$ is the open state, $C$ is the closed state.

According the principle of mass conservation $ C + O = 1 $ where $ C $ denotes the closed state

"From here, it can simply shown that under steady-state conditions that $O_{\inf} = \frac{k_1}{k_1 + k_{-1}}$

and the relaxation time constant of the reaction $\tau = \frac{1}{k_1 + k_{-1}} $ "

My question is, how are $O_{\inf}$ and $\tau$ derived?

Answer 1

These are really math problems (algebra and basic calculus) rather than biology problems.

Oinf comes from solving algebraically the first equation you posted for steady state: i.e., when dO/dt = 0; just solve for O.

The time constant comes because this is a first-order exponential decay, which you get when you solve an equation (calculate an integral) of the form dO/dT = -lambda * O. The solution to that equation is an exponential decay of the form O(t) = O(0) * exp(-lambda*t)

tau is defined as 1/lambda and gives you the decay rate(relaxation time constant).

Answer 2

My question is, how are $O_{inf}$ and $\tau $ derived?

".. it can simply be shown that under stead-state conditions .. " -- By specifically keeping in mind steady-state conditions, certain assumptions can be made when utilizing the mathematical model that the book proposes, which will ultimately allow for us to arrive at the conclusions regarding $O_{inf}$ and $\tau$.

---

To derive $O_{inf} = \frac{k_{1}}{k_{1} + k_{-1}}$

We'll utilize the differential equation that was provided by the book, and set $\frac{\mathrm{dO} }{\mathrm{d} t} = 0$, given the fact that we're considering steady-state conditions; i.e., a state of the ion channel in which its voltage charge and discharge have the same rate. By forcing the change in voltage charge & discharge to have a value of zero, we can solve for the steady-state voltage charge, represented by $O$.

$$\frac{\mathrm{dO} }{\mathrm{d} t} = 0 = k_{1}(1-O)-k_{-1}O$$

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: = k_{1} - k_{1}O + k_{-1}O$$

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: = k_{1} - O(k_{1} + k_{-1})$$
We'll now isolate $O$ by moving its terms to the L.H.S. of the equation, and then dividing by $(k_{1} - k_{-1})$.

$$0 = k_{1} - O(k_{1} + k_{-1}) $$

$$O(k_{1} + k_{-1}) = k_{1} $$

$$O_{inf} = \frac{k_{1}}{k_{1} + k_{-1}} $$

Given that the book mentions conservation of mass when contextualizing the expression $C + O = 1$, it would seem that the result of this (mathematical) expression quantifies open (and close) state of an ion channel in terms of the number of ions that are not reinforcing the conductance state at a given time. I feel that this distinction is important to make because I've encountered this equation within other contexts, and each context brings with it different sets of assumptions that can and can't be made.

---

To derive $\tau = \frac{1}{k_{1} + k_{-1}}$

Again, we start with the differential equation, but this time we'll integrate. For ease of notation, we'll first make the substitutions $O = y$ and $\frac{\mathrm{dO}}{\mathrm{d} t} = y'$.

Beginning with an intermediate step from the previous section,

$$\frac{\mathrm{dO} }{\mathrm{d} t} = k_{1} - O(k_{1} + k_{-1})$$

$$y' = k_{1} - y(k_{1} + k_{-1})$$

and now applying separation of variables,

$$\frac{y'}{k_{1} - y(k_{1} + k_{-1})} = 1$$

We then use $u$-substitution to integrate the L.H.S. of the equation, where $u = k_{1} - y(k_{1} + k_{-1})$ and $u' = -y'(k_{1} + k_{-1})$, which yields

$$ \int \frac{y'}{k_{1} - y(k_{1} + k_{-1})} = \int 1$$
$$-\frac{1}{k_{1} + k_{-1}} \: \int \frac{u'}{u} = \int 1$$
$$-\frac{ln|u|}{k_{1} + k_{-1}} = t$$

Solving for $u$, we have

$$- \: \frac{ln|u|}{k_{1} + k_{-1}} = t$$
$$ln|u| = \: - \: t(k_{1} + k_{-1})$$
$$u = exp\begin{Bmatrix} - t \: (k_{1} + k_{-1}) \end{Bmatrix}$$ which is the same as $$k_{1} - y(k_{1} + k_{-1}) =exp \begin{Bmatrix} - t \: (k_{1} + k_{-1}) \end{Bmatrix}$$

Now, solving for $y$ and back-substituting $O$ for $y$,
$$O = \frac{k_{1}}{k_{1} + k_{-1}} \: \: exp \begin{Bmatrix} - t \: (k_{1} + k_{-1}) \end{Bmatrix}$$
and noticing that $\frac{k_{1}}{k_{1} + k_{-1}} = O_{inf}$ we have

$$O = O_{inf} \: \: exp \begin{Bmatrix} - t \: (k_{1} + k_{-1}) \end{Bmatrix}$$
Lastly, we re-express the exponential argument as

$$- t \: (k_{1} + k_{-1}) = \frac{-t}{\frac{1}{k_{1} + k_{-1}}}$$
where $\tau = \frac{1}{k_{1} + k_{-1}},$ to then get

$$O = O_{inf} \: \: e^{-t/\tau}$$

which fits the form of the exponential decay equation, $N(t) = N_{0} \: e^{-t/\tau}$, where $N = O$ and $N_{0} = O_{inf}$, and $\tau$ is defined to be the average length of time that an ion remains unchanged by the conductance state of an ion channel at a given time $t$.